This is a bit of a detective story, a bit of a puzzle. The method is archaeological.
This morning a colleague of mine sends me an e-mail entitled “importance of correlations.” There are several attachments. One of them is an article by Don Chance entitled “Rethinking Implied Volatility” (*). In it, the author writes the following about the Black-Scholes model for pricing financial options:

“As we said earlier, the Black-Scholes model produces implied volatilities of traded options that can vary by exercise price for a given underlying asset. How should we respond to such a finding? First, we could suggest that the Black-Scholes model is not correct. Since there cannot be more than one volatility of the underlying asset, the model must be incorrect. Case closed.”

I reply to my colleague’s e-mail, saying this:

“On the incorrectness of Black-Scholes and on the “smile” in particular (different “implied volatilities” for different strikes), I had shown on a few examples – and back in the early nineties (I should have the unpublished paper somewhere) that the smile vanishes if you split the “implied volatility” into two components a “true” implied volatility and an additional variable: the option writer’s “profit margin” – constant across strikes and easily calculated as the value that removes the smile, i.e. returns a single – correct – implied volatility.”

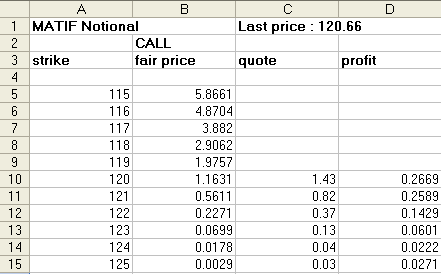
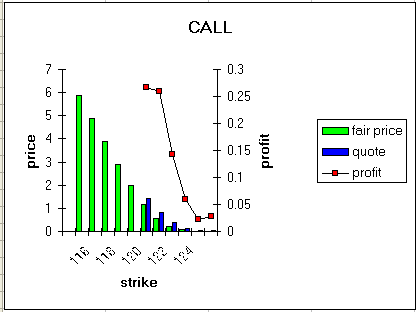
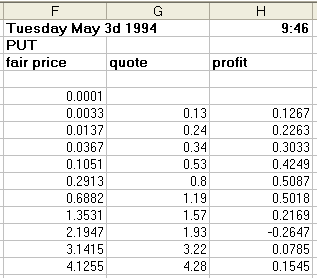
Back home, I look for that paper: I remember it was short: 2 to 3 pages, with diagrams, I can’t find it. The only thing I can find is an Excel spreadsheet with data and the diagrams. Here they are.

I find also a reference to my piece of research in a lecture I gave at ABN Amro in Amsterdam in November 1996. Here is the relevant passage:

“Actually, the fair prices calculated this way (**) are lower than those observed on the markets. This is however the case as well for fair prices calculated according to the theoretical models of Black-Scholes and Cox-Ross-Rubinstein. Prices closer to actual market prices obtain if one adds a particular constant to every one of them. This additional constant can be considered as the profit of the seller or writer of options: to his/her objective loss expectancy s/he adds a margin of actual profit. This last parameter has been overlooked in all theoretical models. When introduced it removes a well-known anomaly of prices generated by the Black-Scholes model, the volatility skew or « smile ».”

————-
(*) Don Chance, Ph.D., CFA, “Rethinking Implied Volatility,” Financial Engineering News, January/February 2003

(**) Sum of potential gains times their probabilities.